Chiral Plasma in the Early Universe: Primordial Magnetic Fields, Instabilities, and Gravitational Wave Production
Content
Introduction to Chiral Plasma in the Early Universe
In the early universe, the dynamics of charged particles in the presence of magnetic fields can give rise to complex phenomena due to the chiral anomaly. Chiral plasma refers to a plasma where the left- and right-handed particles behave differently due to the breaking of parity symmetry. These dynamics are significant because they affect the generation and evolution of magnetic fields and play a role in the production of gravitational waves.The interactions between chiral fermions and gauge fields (like the electromagnetic field) during the early stages of cosmic evolution can amplify primordial magnetic fields and induce instabilities known as chiral plasma instabilities.
Key questions:
- How do chiral effects modify the evolution of the early universe?
- What role do they play in cosmic magnetic field generation?
- How do they affect the evolution of gravitational waves?
Theoretical Background
Chiral Anomaly
In a quantum field theory framework, the chiral anomaly occurs when a classical symmetry (like chiral symmetry) is broken by quantum effects. Specifically, in the presence of a magnetic field, the number of left- and right-handed particles is not conserved. This leads to the creation of a chiral current, which in turn affects the evolution of the magnetic field.The chiral anomaly can be described by the equation:
To write a detailed report on chiral plasma in the early universe, you can structure it as follows, covering both the physical intuition and mathematical formalism. Here is a comprehensive outline with explanations: \[ \partial_\mu J^\mu_5 = \frac{e^2}{16\pi^2} \epsilon^{\mu\nu\rho\sigma} F_{\mu\nu} F_{\rho\sigma} \] where \( J^\mu_5 \) is the axial current, \( e \) is the electric charge, and \( F_{\mu\nu} \) is the electromagnetic field strength tensor. The term on the right-hand side describes the breaking of chiral symmetry due to the electromagnetic field.Chiral Magnetic Effect (CME)
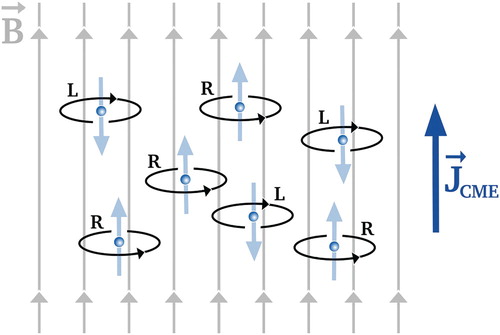
One of the most important consequences of the chiral anomaly in a plasma is the chiral magnetic effect (CME). The CME describes the generation of an electric current along the direction of an applied magnetic field in a plasma with unequal populations of left- and right-handed fermions. The current \( \vec{J} \) is proportional to the chiral chemical potential \( \mu_5 \): \[ \vec{J} = \frac{e^2 \mu_5}{2\pi^2} \vec{B} \] where \( \vec{B} \) is the magnetic field, and \( \mu_5 = \mu_R - \mu_L \) is the chiral chemical potential, which represents the imbalance between the number densities of right-handed and left-handed fermions. This effect plays a key role in the amplification of primordial magnetic fields in the early universe.Chiral Plasma Instabilities in the Early Universe
Chiral Plasma Evolution
The chiral plasma in the early universe is governed by the interaction between chiral fermions and gauge fields. When a chiral imbalance exists (i.e., \( \mu_5 \neq 0 \)), the chiral magnetic effect can lead to the growth of instabilities in the magnetic field. These chiral plasma instabilities occur because the presence of the chiral magnetic current \( \vec{J} \) feeds back into the evolution of the magnetic field. The evolution of the magnetic field and the chiral chemical potential can be described by the coupled differential equations: \[ \frac{\partial \vec{B}}{\partial t} = \nabla \times \left( \vec{v} \times \vec{B} - \eta \nabla \times \vec{B} \right) + \frac{e^2 \mu_5}{2\pi^2} \nabla \times \vec{B} \] \[ \frac{\partial \mu_5}{\partial t} + \nabla \cdot \vec{J}_5 = -\frac{e^2}{8\pi^2} \vec{E} \cdot \vec{B} \] where \( \vec{v} \) is the plasma velocity, \( \eta \) is the magnetic diffusivity, and \( \vec{E} \) is the electric field. The term \( \vec{E} \cdot \vec{B} \) in the second equation represents the energy transfer between the electromagnetic field and the chiral chemical potential, leading to the growth of magnetic helicity (twisting of magnetic field lines).Magnetic Field Generation via Chiral Instabilities
The presence of a chiral plasma instability in the early universe can lead to the generation of strong **primordial magnetic fields**. These fields could be the seeds for the large-scale magnetic fields observed in galaxies and galaxy clusters today.Evolution of the Magnetic Field
The energy stored in the chiral imbalance is converted into magnetic energy through the chiral magnetic effect, causing the magnetic field to grow exponentially. This can be analyzed by solving the coupled equations for \( \vec{B} \) and \( \mu_5 \). In the early universe, the growth rate of the magnetic field is determined by the initial chiral chemical potential \( \mu_5 \). The magnetic field grows until the chiral imbalance is depleted, after which the magnetic field reaches saturation. The growth of magnetic field strength can be roughly estimated as: \[ B(t) \sim B_0 \exp\left( \frac{e^2 \mu_5 t}{2\pi^2} \right) \] where \( B_0 \) is the initial seed magnetic field, and \( t \) is the time scale.Chiral Magnetic Instability Saturation
As the magnetic field grows, it eventually saturates when the chiral chemical potential \( \mu_5 \) is sufficiently depleted. At this stage, the chiral anomaly ensures that the system reaches a quasi-equilibrium state, with magnetic helicity conserved and the plasma becoming nearly isotropic.Chiral Plasma and Gravitational Waves
The dynamics of a chiral plasma in the early universe can also produce primordial gravitational waves. These waves arise due to the coupling between the magnetic field and the chiral current, which leads to anisotropic stresses in the plasma. These stresses source tensor perturbations in the metric, producing gravitational waves.Tensor Perturbations from Chiral Instabilities
The Einstein field equations in the presence of a magnetized chiral plasma can be written as: \[ R_{\mu\nu} - \frac{1}{2} g_{\mu\nu} R = 8\pi G T_{\mu\nu} \] where \( T_{\mu\nu} \) includes contributions from the magnetic field and the chiral current. The anisotropic stress due to the magnetic field sources tensor perturbations, which propagate as gravitational waves. The spectrum of these gravitational waves depends on the scale of the magnetic field and the strength of the chiral imbalance, and they could potentially be observed by future gravitational wave detectors.Conclusion and Implications for Cosmology
The study of chiral plasma in the early universe has profound implications for both cosmology and astrophysics. The generation of primordial magnetic fields through chiral plasma instabilities could explain the large-scale magnetic fields observed today. Additionally, the production of gravitational waves from these instabilities offers a potential observational signature that could be detected by upcoming experiments. The key takeaways from the study of chiral plasma in the early universe are:- The chiral magnetic effect plays a central role in amplifying primordial magnetic fields.
- Chiral plasma instabilities lead to the exponential growth of these fields until saturation.
- These instabilities may also produce primordial gravitational waves, contributing to the cosmic gravitational wave background.
References
- Primordial Magnetic field and kinetic theory with Berry curvature, Jitesh R. Bhatt, Arun Kumar Pandey [arXiv:1503.01878 [astro-ph.CO]] Phys.Rev.D. 94, 043536
- Primordial Generation of magnetic field, Jitesh R. Bhatt, Arun Kumar Pandey [arXiv:1507.01795 [gr-qc]] Springer Proc.Phys. 174 (2016) 409-413
- Effect of background magnetic field on the normal modes of conformal dissipative chiral hydro and a novel mechanism for explaining pulsar kicks Arun Kumar Pandey, Manu George [arXiv:1609.01848 (astro-ph.CO)]
- Chiral Battery, scaling laws and magnetic fields, Sampurn Anad, Jitesh R. Bhatt & Arun Kumar Pandey [arXiv:1705.03683 (astro-ph.CO)] JCAP, JULY 2017
- Chiral Plasma Instability and Primordial Gravitational waves, Sampurn Anad, Jitesh R. Bhatt & Arun Kumar Pandey [arXiv:1801.00650 [astro-ph.CO] (2019)] Eur. Phys. J. C (2019) 79: 119.
- Viscosity in cosmic fluids, Jitesh R Bhatt, Pravin Kumar Natwariya, Arun Kumar Pandey, arXiv:1907.03445 [astro-ph.CO] (2019) Eur. Phys. J. C 80 (2020) 8, 767
- Magnetic fields in a hot dense neutrino plasma and the Gravitational Waves Arun Kumar Pandey, Pravin Kumar Natwariya, Jitesh R Bhatt, arXiv:1911.05412 [astro-ph.CO] (2020), Phys. Rev. D 101, 023531 (2020)
- Gravitational waves in neutrino plasma and NANOGrav signal, Arun Kumar Pandey arXiv:2011.05821 [astro-ph.CO] (2020) [Eur.Phys.J.C 81 (2021) 5, 399]
- Generating Seed magnetic field à la Chiral Biermann battery, Arun Kumar Pandey, Sampurn Anand (Phys. Rev. D. 104, 063508 (2021))
Some other interesting things to know:
- Visit my website on For Data, Big Data, Data-modeling, Datawarehouse, SQL, cloud-compute.
- Visit my website on Data engineering